
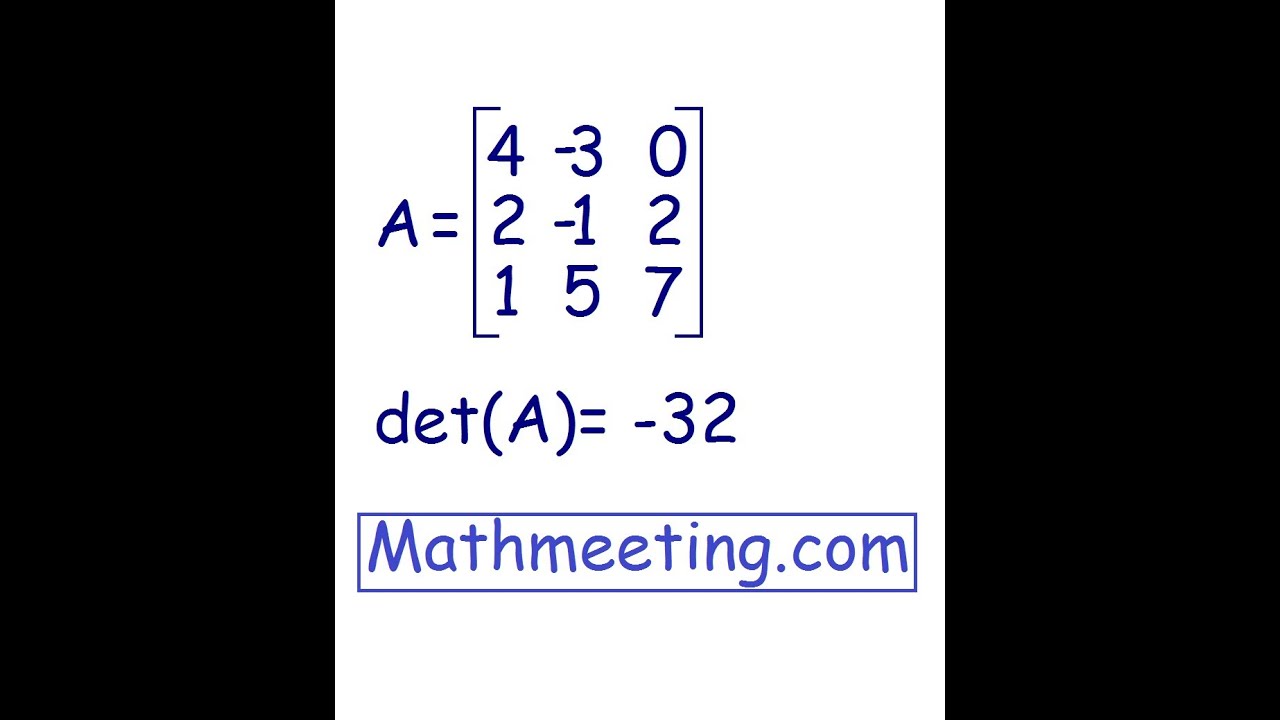
Using these operations, a matrix can always be transformed into an upper triangular matrix, and in fact one that is in row echelon form. Adding a multiple of one row to another row.There are three types of elementary row operations: To perform row reduction on a matrix, one uses a sequence of elementary row operations to modify the matrix until the lower left-hand corner of the matrix is filled with zeros, as much as possible. The method is named after Carl Friedrich Gauss (1777–1855). This method can also be used to compute the rank of a matrix, the determinant of a square matrix, and the inverse of an invertible matrix. It consists of a sequence of operations performed on the corresponding matrix of coefficients. In mathematics, Gaussian elimination, also known as row reduction, is an algorithm for solving systems of linear equations.
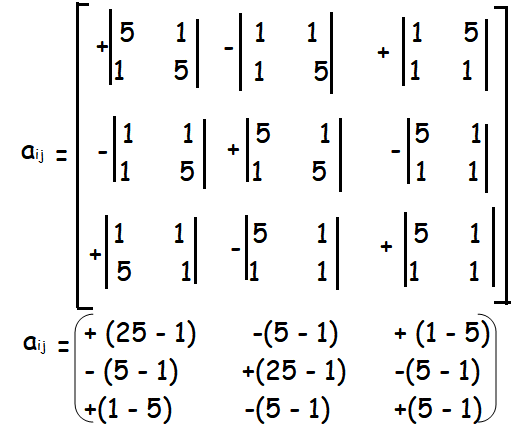
Last Step: Use row replacement to clear all entries above the pivots, starting with the last pivot.Algorithm for solving systems of linear equations.Step 3a: Swap the 3rd row with a lower one so that the leftmost nonzero entry is in the 3rd row.Step 2c: Use row replacement so all entries below this 1 are 0.Step 2b: Scale the 2nd row so that its first nonzero entry is equal to 1.Step 2a: Swap the 2nd row with a lower one so that the leftmost nonzero entry is in the 2nd row.Step 1c: Use row replacement so all entries below this 1 are 0.Step 1b: Scale the 1st row so that its first nonzero entry is equal to 1.Step 1a: Swap the 1st row with a lower one so a leftmost nonzero entry is in the 1st row (if necessary).We will not prove uniqueness, but maybe you can! Algorithm (Row Reduction) This assumes, of course, that you only do the three legal row operations, and you don’t make any arithmetic errors. The uniqueness statement is interesting-it means that, no matter how you row reduce, you always get the same matrix in reduced row echelon form. We will give an algorithm, called row reduction or Gaussian elimination, which demonstrates that every matrix is row equivalent to at least one matrix in reduced row echelon form. Subsection 1.2.3 The Row Reduction Algorithm TheoremĮvery matrix is row equivalent to one and only one matrix in reduced row echelon form. This is one possible explanation for the terminology “pivot”. We used the pivot position in the matrix in order to make the blue line pivot like this. We can think of the blue line as rotating, or pivoting, around the solution ( 1,1 ). What has happened geometrically is that the original blue line has been replaced with the new blue line y = 1. Scaling: we can multiply both sides of an equation by a nonzero number.There are three valid operations we can perform on our system of equations: In other words, we will combine the equations in various ways to try to eliminate as many variables as possible from each equation. We will solve systems of linear equations algebraically using the elimination method. Subsection 1.2.1 The Elimination Method ¶ In this section, we will present an algorithm for “solving” a system of linear equations. Vocabulary words: row operation, row equivalence, matrix, augmented matrix, pivot, (reduced) row echelon form.Learn which row reduced matrices come from inconsistent linear systems.

Learn to replace a system of linear equations by an augmented matrix.Hints and Solutions to Selected Exercises.3 Linear Transformations and Matrix Algebra


 0 kommentar(er)
0 kommentar(er)
